Galileo’s Vision

It is a very beautiful thing, and most gratifying
to the sight, to behold the body of the moon,
distant from us almost sixty earthly radii, as if
it were no further away than two such measures–
so that its diameter appears almost thirty times
larger, its surface nearly nine hundred times,
and its volume twenty-seven thousand times
as large as when viewed with the naked eye.
In this way one may learn with all the certainty
of sense evidence that the moon is not robed
in a smooth and polished surface but is in fact
rough and uneven, covered everywhere, just like
the earth’s surface, with huge prominences,
deep valleys, and chasms.

A thirty power telescope (comparable to the most common of field glasses which are 35 power) reveals not just a thirty-fold enlargement, but a 900-fold enlargement of areas, and a 27,000-fold enlargement of volume. We no doubt recall that area increases as the square of a diameter, and volume as a cube, but Galileo may help us to inform our sight through his attention to such ratios, to enable us to see with heightened appreciation the moon our field glasses approach. If the moon is sixty earth diameters away, the moon's image through a thirty-power lens can appear to an informed viewer two earthly radii away. How are we to see this moon through Galileo’s eyes?

Consider the sensational appearance of a total eclipse of the sun. Annie Dillard reminds us that a total eclipse we see is far from total: the eclipsed sun will reappear minutes later, restoring us to light, to warmth, to normalcy. But eventually, when the sun consumes its supply of fuel, it will burn out. That total eclipse will leave earth without light or energy, a domain dark, cold and barren. Galileo’s viewing of the moon evokes his vision of a rocky world, devoid of life or growth, where the presence of a single orange on a single orange tree would be a miracle all but unimaginable were an imaginable imaginer to exist in such circumstances.  We have, indeed, a rocky moon, We have, indeed, a sun which consumes finite energy thereby radiating light and warmth, the preconditions for metabolism and replication and consciousness, for life as we know it.

Total Eclipse

Consider the diameter of the earth. How may we consider this measure? Eratosthenes, living in the early centuries of the common era, exercised his curiosity with sufficient success to find himself the director of the library at Alexandria, where every manuscript found on every passing ship was copied, and the library grew as each copy accumulated. Discovering a story that in one place, at one time, light shone to the bottom of a deep well, Eratosthenes for the first time in recorded history intuited the size of the earth.

In Cyrene, now the site of Aswan, so the story went, on the summer solstice, light reached the bottom of a deep well. This light, Eratosthenes understood, came to earth directly overhead. Eratosthenes, of course, understood seasons at Alexandria. He understood that societies could elaborate as many seasons as they wished, but all such seasons to work would depend on two celestial events:  at noon, on two days of the year the sun will be either highest in the sky, or lowest in the sky. We call the day with most light and warmth the summer solstice, and we call the day with the least light and warmth the winter solstice. Summer is relatively light and hot, winter is relatively dark and cold. We also take the equinoxes, the days exactly between solstices, as seasonal markers, spring and fall. But further divisions are possible. Eratosthenes also noted a crucial happenstance: Cyrene was almost due south of Alexandria.

Eratosthenes understood that he never experienced the sun overhead at noon. Even on the longest day of the year (the summer solstice) The sun rose a bit less than overhead. His discovery of the size of the earth required merely placing a pole upright in the ground and monitoring the shadow cast by the sun. As the sun appeared higher in the sky, the shadow shrunk. Eventually as the sun declines, the shadow will grow. At the decisive moment, noon, Eratosthenes measured the angle between stick and shadow when the sun was almost overhead. His measurement was 7.2 degrees, one fiftieth of a full circle. By measuring the distance between Alexandria and Cyrene, Eratosthenes would measure one fiftieth of the diameter of the earth. Whether he used chains or revolutions of a wheel, whatever the actual measure of stadia, his unit of measure, few doubt that his distance approached five-hundred miles. Five-hundred times fifty measures from Alexandria to Cyrene traversed the earth, a great circle of 25,000 miles.

Galileo assumes the moon is two-sevenths the diameter of the earth.  His estimate of the earth’s diameter was 7,0000 miles (less accurate than Eratosthenes). His estimate of the distance of the moon from earth is sixty earthly radii, in his measure (60x3500), or 210,000 miles. Given earth’s radius as 8,000 miles, the moon’s distance will be 240,000 miles.

Far more significant than departures from modern evaluations is Galileo’s formula: 60 earthly radii. If the moon is 2/7 the diameter of the earth, and the shadow of the earth form the sun (congruent with the shadow of a quarter just covering the sun from a viewer’s eyes), we may trace his discovery to get moonlight into the chamber of our imagination.

1

Hold a quarter, one inch in diameter, out at arm’s length, towards the sun. The quarter will not fully block the sun’s rays. But extend the quarter further, and at some point it will just eclipse the sun. If the quarter is one inch, the distance will be 109 inches.

2

Consider a solar eclipse: when the moon fully blocks the sun, the corona reveals that the moon’s apparent size is almost exactly equal to the sun’s apparent size. The apparent sun and the apparent moon inhabit a cone exactly equal in angle to that cast by our quarter. Looking out from earth imagine three objects: the quarter, the moon and the sun. During a solar eclipse you can see behind your quarter the moon blocking sunlight and around the moon the coronal flares of the distant sun.

3

Now consider a lunar eclipse. The moon first touches the shadow of the earth at a precise time. The moon’s trailing edge will disappear at a precise time. Eventually the leading edge of the moon will just appear some precise time later. Reproducible measurements show that the total time the moon is in eclipse allows for passage of 2 1/2 times the diameter of the moon. Whatever time the moon’s disappearance takes, the reappearance occurs in 2.5 times that time.

4

Now imagine  the full moon just before it enters the earth’s shadow. From earth, the angle of view will exactly equal that defining the earth's shadow (or that formed between eye and quarter held to the sun). If 2 ½ moons pass though the earth’s shadow, a full moon also appears below these moons. Since the angle of the new moon from
earth exactly equals the angle at the tip of the earth’s shadow, the outer rays are parallel. 3 ½ moons equal the earth’s diameter. If the earth’s diameter is 7,000 miles, the moon’s diameter is 2,000 miles.

Consider reversing the position of earth and moon to confirm equal angles, and to see that the outer rays must be parallel:

5

If the earth’s shadow is 109 times the diameter of the earth, it is also 218 times the radius of the earth. And if the moon’s radius is 2/7 that of the earth, then the distance of the moon from earth is 2/7 x 218 x the earth’s diameter, just over 60 earthly radii.

Now let’s consider mountains on the moon. Assume the radius of the moon to be 1,000 miles (2/7 the radius of the earth (for Galileo the earth’s diameter is 3,500 miles).
A spot of light in the dark quadrant of the moon appears
consistently to Galileo as 1/20^th the distance of the diameter, or 100 miles. Since the radius of the moon according to Galileo is 1000 miles, and the distance to the spot from the horizontal is 100 miles, we can use the Pythagorean theorem to  find the distance from the center of the moon to the spot of light. The distance 1004 exceeds the radius by 4 miles. A mountain four miles high reaches just high enough to capture and reflect the light streaming over the top of the moon.

Galileo calls attention to the light capturing a mountain peak rising up from the moon's shadow to reach sunlight just passing over the moon, to the light as it spreads until peak and valley are fully illuminated, just as light on earth first strikes a peak, and then as the sun rises spreads down into the valley below.:

Again, not only are the boundaries of shadow and light in the moon seen to be uneven and wavy, but still more astonishingly many bright points appear within the darkened portion of the moon, completely divided and separated from the illuminated part and at a considerable distance from it. After a time these gradually increase in size and brightness, and an hour or two later they become joined with the rest of the lighted part which has now increased in size. Meanwhile more and more peaks shoot up as if sprouting now here, now there, lighting up within the shadowed portion; these become larger, and finally they too are united with that same luminous surface which extends ever further. An illustration of this is to be seen in the figure above. And on the earth, before the rising of the sun, are not the highest peaks of the mountains illuminated by the sun’s rays while the plains remain in shadow? Does not the light go on spreading while the larger central parts of those mountains are becoming illuminated? And when the sun has finally risen, does not the illumination of plains and hills finally become one?

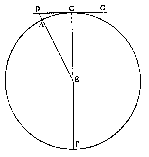
Since according to very precise observations the diameter of the earth is seven thousand miles, CF will be two thousand, CE one thousand, and one-twentieth of CF will be one hundred miles. Now let CF be the diameter of the great circle which divides the light part of the moon from the dark part (for because of the very great distance of the sun from the moon, this does not differ appreciably from a great circle), and let the moon, this does not differ appreciably from a great circle), and let A be distant from C by one-twentieth of this. Draw the radius EA, which, when produced, cuts the tangent line GCD (representing the illuminating ray) in the point D. Then the arc CA, or rather the straight line CD, will consist of one hundred units whereof CE contains one thousand, and the sum of the squares of DC and CE will be 1,010,000. This is equal to the square of DE; hence ED will exceed 1,004, and AD will be more than four of those units of which CE contains one thousand. Therefore the altitude AD on the moon, which represents a summit reaching up to the solar ray GCD and standing at the distance CD from C, exceeds four miles.

Appropriate surprise can mark the intersection of intuited beauty and informed geometry. Howard Nemerov incorporates Galileo's visionary powers to recognize seven blue swallows . . .

Blue Swallows